1- Calculons Qri:
Qri=[Ni(aq)2+]i[Co(aq)2+]i=C1C2=3,0⋅10−20,3=10
Qr <K, donc le système évolue dans le sens (1) : le sens qui correspond à la réduction de Ni(aq) 2+ et l'oxydation anodique de Co(s).
Donc l'électrode de cobalt constitue l'anode (la borne (−) de la pile) et l'électrode de nickel constitue la cathode (la borne (+) de la pile).
la réponse juste est donc:
d - Le sens du courant électrique à l'extérieur de la pile est de l'électrode de nickel vers l'électrode de cobalt.
2.
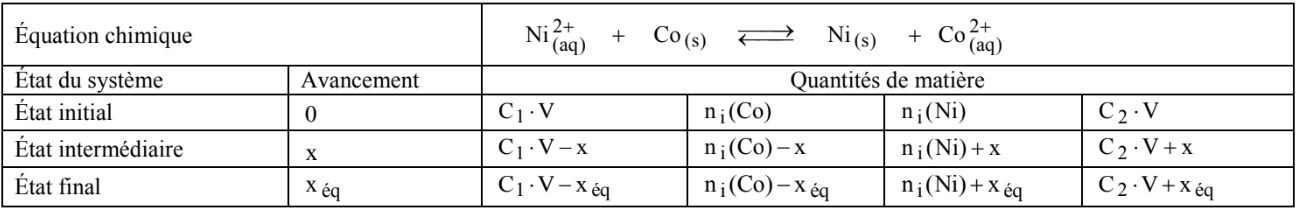
K=[Ni(aq)2+]eˊq [Co(aq)2+]eˊq =VC1⋅V−xeˊq VC2⋅V+xeˊq =C1⋅V−xeˊq C2⋅V+xeˊq ⇒xeˊq = K+1K⋅C1−C2⋅V
À la date te, la quantité de matière échangée est : n(e−)=FI⋅te=2xeˊq
FI⋅te=2xeˊq=2 K+1K⋅C1−C2⋅V⇒te=(K+1)⋅I2( K⋅C1−C2)⋅V⋅F
te=(100+1)×0,12(100×0,03−0,3)×0,100×96500=5159,4 s≈1 h26 min
3. Δm=Δn(Ni)⋅M(Ni)
avec Δn=xeˊq = K+1K⋅C1−C2⋅V
⇒Δm= K+1K⋅C1−C2⋅V⋅M(Ni)
Δm= K+1K⋅C1−C2⋅V⋅M(Ni)=101100×0,03−0,3×0,100×58,7≈0,157 g
Remarque : on peut écrire : xeˊq =2n(e−)=2 FI⋅te
⇒Δm=xeˊq ⋅M(Ni)=2 FI⋅te⋅M(Ni)=2×965000,1×5159,4×58,7≈0,157 g