1. La valeur de la poussée est égale au poids du volume de fluide (l'air) déplacé :
FA=ρ2⋅V⋅g
2. {P=m⋅g=ρ1⋅V⋅gFA=ρ2⋅V⋅g⇒FAP=ρ2ρ1=1,3103=103×0,77=770
Le poids est environ 800 fois supérieur à la poussée d'Archimède ; celle-ci peut être négligée devant le poids.
3.1. La goutte est soumise à son poids et à la force de frottement. Écrire la seconde loi de Newton sur un axe vertical orienté vers le bas :
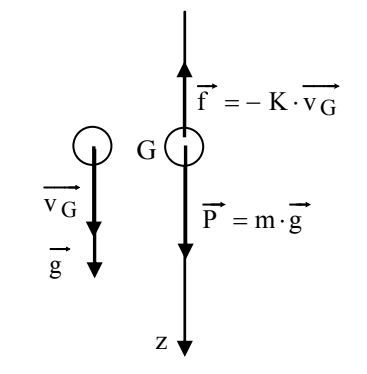
P+f=m⋅g
m⋅g−K⋅vG=m⋅dtdvG
⟶m⋅g−K⋅vG=m⋅dtdvG
⟶dtdvG=−mK⋅vG+g⟶{A=−mKB=g
3.2. (B) a la dimension d'une accélération (m⋅s−2)
(A⋅vG) a la dimension d'une accélération et vG celle d'une vitesse (m⋅s−1).
(A) est donc l'inverse d'un temps (s−1).
3.3.1. On a :
t=3,4 s⟶ aG=dtdvG=A⋅vG+B=−3,24⋅10−1×21,0+10=3,196 m⋅s−2≈3,2 m⋅s−2
3.3.2 aG(ti)=ΔtvG(ti+1)−vG(ti)
⇒vG(ti+1)=aG(ti)⋅Δt+vG(ti)
⇒vG(3,6 s)=aG(3,4 s)⋅Δt+vG(3,4 s)=3,196×0,2+21,0=21,6392 m⋅s−1≈21,6 m⋅s−1
3.3.3. Le pas doit être petit. Généralement, on prend Δt=10τ ( τ est le temps caractéristique du mouvement)
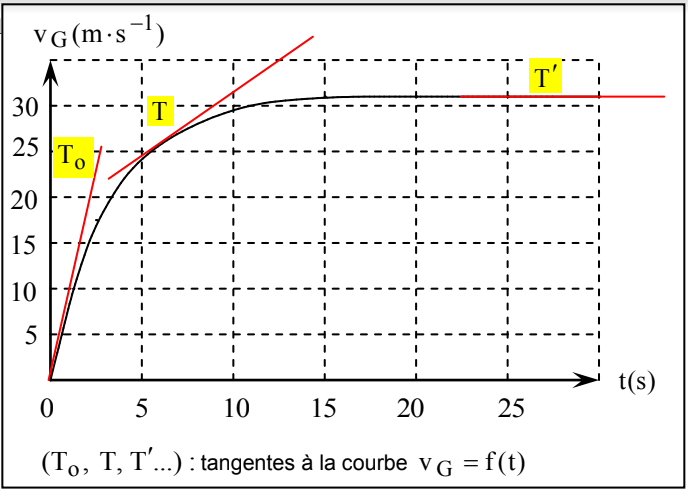 3.4.1. L'accélération est égale au coefficient directeur de la tangente à la courbe à une date donnée.
3.4.1. L'accélération est égale au coefficient directeur de la tangente à la courbe à une date donnée.
Or ces tangentes sont de moins en moins inclinées sur l'horizontale.
L'accélération diminue au cours du temps.
3.4.2. En régime permanent, le mouvement est rectiligne uniforme et l'accélération est nulle.
3.4.3 m⋅g−K⋅vGlim=m⋅dtdvGlim=0
K⋅vGlim=m⋅g vGlim=Km⋅g